
|
Within two months of the discovery, Challis, Airy and James Glaisher, three of Britain’s top astronomers, had publicly committed themselves to the radically unsound view that Adams and Leverrier’s predictions agreed ‘within one degree.’ Nothing has more skewed the debate than this claim: they differed by about four degrees. As Challis was scouring the sky at nine arcminute intervals, this difference was substantial. The only thing within one degree was Leverrier’s prediction.
Accuracy of Four Historic Solutions Compared, at the Neptune-Discovery Date
Leverrier’s accuracy increased
several-fold, between his first and second prediction, whereas Adams
decreased his between his Hyp I (supposedly, October 1845) and Hyp.
II (Sept 2nd 1846). The Table has a line giving ‘error’
in degrees and minutes. A reconstruction of Leverrier’s computations
was performed by Baghdady (1980) in a PhD, who solved Leverrier’s
Lagrangian perturbation-equations to a higher order of accuracy
and obtained a final error in celestial longitude of merely sixteen
arcminutes, as compared with the fifty-two arcminutes error of the
historical prediction - sterling evidence that Leverrier’s
prediction really was the brilliant feat of deductive logic which
he believed it was, and not just a coincidence as is sometimes alleged
(The computations by Perceval Lowell as led to the discovery of
Pluto, were of this latter kind). Distance Estimates
Neither could decrease their mean radius any further, because at around 35.3 AU a resonance-instability effect appeared, due to a 2:5 ratio between their orbit-periods, unknown to either of them, as caused a discontinuity making their computations go haywire. The US astronomer Benjamin Peirce of Harvard pointed out this strange instability in the maths: ‘The distance of 35.3 then is a complete barrier to any logical deduction, and the investigations with regard to the outer space cannot be extended to the interior’(p.443 of Amer. Jnl Sci & Arts 1847; Hubbell & Smith JHA 1992 p.271).But, the perturbation-maths tended to work better at a closer orbit, as Neptune is at 30 AU, defying ‘Bode’s Law’. Both mathematicians circumvented this problem by using a hugely exaggerated eccentricity with the planet near to its perihelion, thereby getting it much nearer than its mean radius.
Co-ordinates Five different co-ordinate systems were used in the Neptune-predictions.
A Twenty-Degree Swing Adams’ preferred solutions swung over a twenty-degree
range from July to September of 1846 - no wonder Challis was unsure
where to point his telescope! We saw above how the July Ephemeris
which he constructed for Challis advocated 336º (see
Adams’ July Ephemeris). Adams’ letter of September
2nd to Airy contained parameters for his ‘Hyp I’ and
‘Hyp II’ versions, but went on to give his preferred
value, always omitted in tellings of the story. His preferred value
came from a further shortening of the mean orbit-radius. We have
seen above how the perturbation-math goes awry when this happens,
due to a resonance-instability that neither Adams nor Leverrier
were in a position to apprehend. Adams decides that he prefers a
mean orbit-radius of 33.6 AU. His mode of expression is a little
opaque. From considering the most recent ‘errors’ i.e.
perturbations of Uranus, One can imagine the terrible temptation to which
Airy became subject. Looking at this letter - the only one Adams
ever sent making a Neptune-prediction, before its discovery - after
the great debacle, with Challis having spent six weeks not finding
a planet which the Germans found in half an hour, and Airy’s
own reputation at stake because he had had set up the clandestine
sky-search ...if only, he mused, one were to ignore the last part
of this letter, stating Adams third and preferred option ... Would
people really notice? Could he stand up before his colleagues and
brazen it out? He decided to take the risk, which proved well justified:
nobody ever did notice. Adams’ a/a1 signifies the helio distance
of Uranus, divided by that of the unknown planet. Nowhere in his
published work does he give a value of the Uranus mean radius, but
taking a likely value will give us 33.6 AU as his final and preferred
radius of the unknown planet. The three mean helio longitudes specified
in this letter, for his close-to-D-day epoch are: 325, 323 and 315
degrees. As we’ve seen, the first two come out at 329 degrees
true helio after applying the Equation of Center. Here however the
eccentricity has become ‘very small.’ That’s all
we are told, so let’s add on one degree for the Equation of
centre. That gives us around 316 degrees, which errs by eleven degrees. After the discovery, Adams wrote uneasily to Airy
about this blunder: ‘In my letter of September 2nd, I inferred
that the mean distance used in my first hypothesis must be greatly
diminished, but I rather hastily concluded that the change in the
mean Long. deduced would be nearly proportional to the change in
the assumed mean distance.’ (15th October ’46). In his
RAS presentation, Adams managed to give himself credit for his shrewd
mean-distance reduction, but without mentioning the large error
into which it led him. Herschel's Portrait courtesy Royal Astronomical Society
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||

 Following
the example of John Herschel in his Outlines of Astronomy (1852),
we compare predictions by expressing them as true heliocentric longitude
at D-day, i.e. September 23rd 1846. (For telescope work one used
geocentric co-ordinates, but calculations are done heliocentrically).
It seems that the perturbation-equations that Adams used produced
initially mean helio longitude (uniformly circular motion), for
a given epoch: Adams used the date of Oct 1st 1845, while Leverrier
computed for Jan 1st, 1847. We must first convert these to ‘D-day’
using a suitable value for mean motion, according to the distance
assumed, then apply the Equation of Centre to get true helio longitude.
(NB, four terms are required in this expansion on account of the
large eccentricities involved: Solar System Dynamics Murray &
Dermott, 1999, p.41)
Following
the example of John Herschel in his Outlines of Astronomy (1852),
we compare predictions by expressing them as true heliocentric longitude
at D-day, i.e. September 23rd 1846. (For telescope work one used
geocentric co-ordinates, but calculations are done heliocentrically).
It seems that the perturbation-equations that Adams used produced
initially mean helio longitude (uniformly circular motion), for
a given epoch: Adams used the date of Oct 1st 1845, while Leverrier
computed for Jan 1st, 1847. We must first convert these to ‘D-day’
using a suitable value for mean motion, according to the distance
assumed, then apply the Equation of Centre to get true helio longitude.
(NB, four terms are required in this expansion on account of the
large eccentricities involved: Solar System Dynamics Murray &
Dermott, 1999, p.41)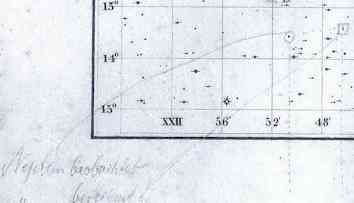 The
Original Bremiker star-map from which Galle and D'Arrest found
Neptune. The two positions marked are where leverrier
predicted it (drawn in on the right) would be and where Neptune
was found (between 52' and 48').
The
Original Bremiker star-map from which Galle and D'Arrest found
Neptune. The two positions marked are where leverrier
predicted it (drawn in on the right) would be and where Neptune
was found (between 52' and 48').